Which Characteristics Best Describe an Equilateral Triangle

All the centres like incentre circumcentre orthocentre centroid the centre of gravity lie at the same point in its interior region. Because it also has the property that all three interior angles are equal it really the same thing as an equiangular triangle.

Equilateral Triangle Definition Properties Formulas Examples

Recall that an obtuse angle is an angle that is greater than 90 degrees and a scalene triangle is a triangle that has all its sides with different lengths and all of its angles with different measures.

. There is an equilateral triangle A B C on each of its inner sides lies N13 points. A triangle with vertices P Q and R is denoted as PQR. Two sides have the same length as each other.

Equilateral triangles are always similar. An equilateral triangle has three sides of equal length and three equal angles. An equilateral triangle is a special type of isosceles so an.

Triangles can be classified in the following three categories based on the length of the sides. A rectangle with all sides equal and four right angles. An equilateral triangle is one in which all three sides are congruent same length.

If we circumscribe a triangle square hexagon etc we draw a circle that surrounds it and touches each of its corners. Its best if they copy down the following information. The proof that the resulting figure is an equilateral triangle is the.

The sum of the length of two sides of a triangle is always greater than the length of the third side. Two significant characteristics of an equilateral triangle are. It is a regular polygon with three sides.

Look at these two examples. Equilateral triangles have no longest and shortest sides. The three internal angles of a triangle always add to 180 degrees.

Two sides meet at a 90 angle. Equilateral Triangle - Triangle with all 3 sides being the same length and angle measurement. The basic formula for calculating the area of equilateral triangle is to multiply the height of the triangle with half of the length of the base.

A triangle has three sides three vertices and three angles. In both methods a by-product is the formation of vesica piscis. A right isosceles triangle has both characteristics.

All angles are less than 90. Right Triangle - Triangle with. It can be proved that all the angles of an equilateral triangle are also equal.

Although this is not the only way to find the area of equilateral triangle and the methodology should be selected according to the characteristics of the triangle. An equilateral triangle can never be obtuse. The area of a triangle is equal to half of the product of its base and height.

The Equilateral triangle shown on the left has three congruent sides and three congruent angles. We know that an isosceles triangle has two sides with equal measure and an equilateral triangle has three equal sides so we can conclude that each equilateral triangle is an isosceles triangle as well. An isosceles triangle has two.

An equilateral triangle is a triangle whose three sides all have the same length. JL is the shortest side so Kis the smallest angle of the triangle. The other side may be shorter or longer in length than the other two sides.

These triangles are scalenes and obtuse at the same time. Define acute obtuse and right triangle. In an equilateral triangle all the sides of a triangle are of equal length.

Circumscribing can be done as a construction. One figure must be a circle. Two sides are equal in length.

An equilateral triangle is simply a specific case of a regular polygon in this case with 3 sides. A triangle with all three sides of equal length. No need to check for any condition of similarity followed by corresponding sides as well.

A parallelogram with all sides equal. The sum of the three interior angles of a triangle is always 180. Triangles are polygons with the least possible number of sides three.

All three angles are congruent and are equal to 60 degrees. If all the sides of a triangle are equal then it is called an equilateral triangle. Therefore an equilateral angle can never be obtuse-angled.

The perpendicular drawn from vertex of the equilateral triangle to the opposite side bisects it into equal halves. A quadrilateral with at least one pair of parallel sides. Find the number of all triangles whose vertices lie at given points on different sides.

The properties of the triangle are that firstly the sum of all the angles of a triangle of all types equals to 1800. PQ QR RP So P Q R An isosceles triangle with only two equal sides can have a longest or a shortest side. All the angles are 60deg.

All equilateral triangles are acute and isosceles. The 90 degree angle will be the angle formed by the sides of equal length. Each angle is 60 degrees.

Since an equilateral triangle has equal sides and angles each angle measures 60 which is acute. An obtuse scalene triangle is a scalene triangle that has an obtuse internal angle. The quadrilateral formed by joining in order the midpoints of the sides of a.

All the facts and properties described for regular polygons. Illustrated definition of Equilateral Triangle. A parallelogram with four right angles.

Each angle is 60. IIAll side lengths are equal. Isosceles Triangles - triangle with 2 equal sides and angle measurement all angles are acute.

In an acute triangle all the angles of a triangle are less than 90 degrees. The angles of the triangle ABC are alpha 35 beta 48. The triangles The triangles ABC and ABC are similar with a similarity coefficient of 2.

A triangle cannot be right-angled and obtuse angled at the same time. Circumscribing can be done as a construction. Properties of Equilateral Triangle All three sides are equal.

An equilateral triangle can be constructed by taking the two centers of the circles and either of the points of intersection. They are the only regular polygon with three sides and appear in a variety of contexts in both basic geometry and more advanced topics such as complex number geometry and geometric inequalities. Secondly the sum of the length of the two sides of a triangle is larger than the length of the third side.

Since a right-angled triangle has one right angle the other two angles. Therefore opposite the three equal sides are three equal angles.

The Equilateral Triangle With A Side Length Equal To 2 Each Of Three Download Scientific Diagram

Types Of Triangles With Examples And Videos Math Projects Life Hacks For School Math Help

Properties Of Equilateral Triangles Brilliant Math Science Wiki

No 5 Equilateral Triangle

What Are The Characteristics Of Equilateral Triangles Mechamath

Inside An Equilateral Triangle There Is A Point Which Is 2 2 And 1 Units Away From The 3 Vertices Respectively What Is This Triangle S Area Quora

What Are The Characteristics Of Equilateral Triangles Mechamath

Inside An Equilateral Triangle There Is A Point Which Is 2 2 And 1 Units Away From The 3 Vertices Respectively What Is This Triangle S Area Quora

Equilateral Triangle Definition Properties Formulas

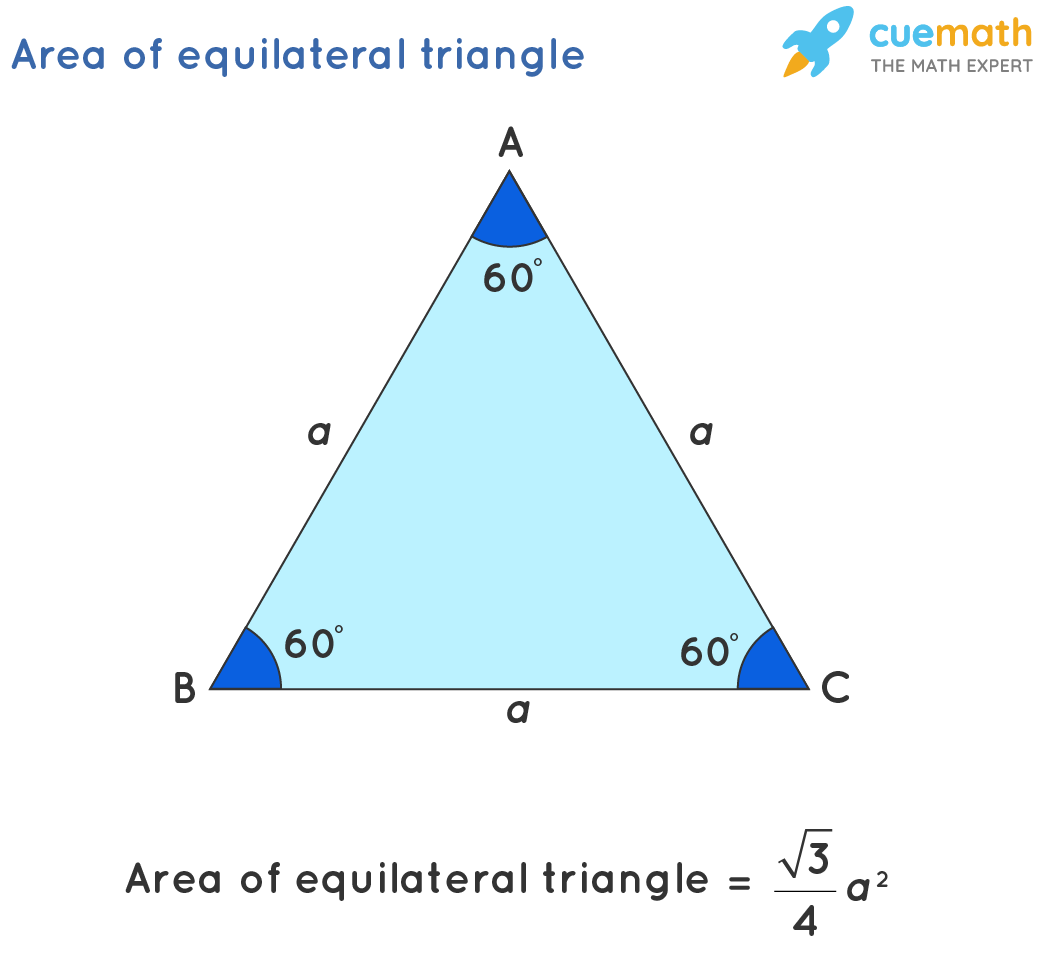
Equilateral Triangle Formula Properties Definition Examples

Poster About Different Types Of Triangles Different Types Of Triangles Math Formula Chart Math Journal

Equilateral Triangle Definition Properties Formulas

Equilateral Triangle Angles Degrees Measurements Video Lesson Transcript Study Com

Triangle Property Foldable For Interactive Notebook Decorating Blogs Interior Wall Paint Gaming Decor

Characteristics Of A Triangle Download Print Worksheet Geometry Worksheets Printable Math Worksheets Worksheets For Kids

Equilateral Triangle Angles Degrees Measurements Video Lesson Transcript Study Com

Equilateral Triangle Definition Properties Formulas Examples

Equilateral Isosceles And Scalene Triangle Basic Geometry Classification Of Triangles Different Types Of Triangles

Equilateral Triangle Formula Properties Definition Examples